
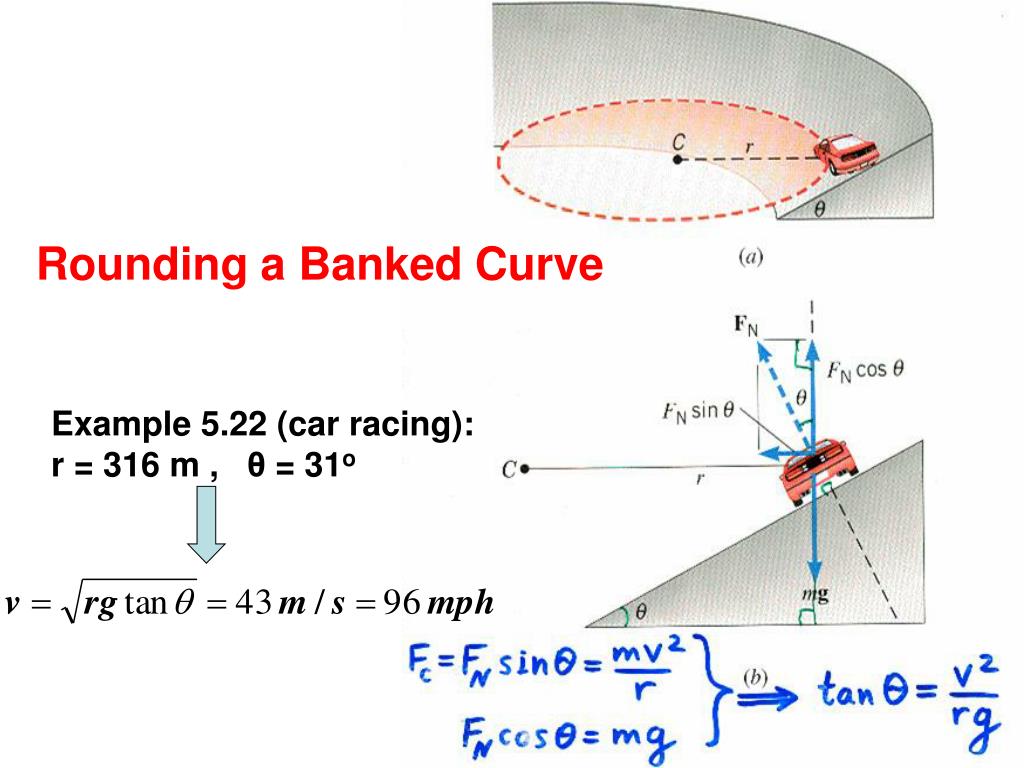
In this case, the acceleration provided by the centripetal force is called the centripetal acceleration. As such, if there are multiple forces acting on an object that is in circular motion, the resultant force should be used.įurthermore, recall that the rate of change of an object’s velocity is also referred to as its acceleration. It can also come from various types of forces, like gravitational force or friction.

This force is always directed towards the centre of the circle. Centripetal force is defined as the net force acting perpendicular to the velocity’s direction. Since an object undergoing motion in a circle is constantly changing direction and hence changing velocity, there must be some force acting on it.
This relationship can be summarised by the following formula: the displacement of the object per unit time, you can simply multiply the angular velocity by the radius of the circle.

Formula: Angular velocity = 2π / Period = 2π x Frequency.
CENTRIPETAL FORCE IN RELATION TO RADIANS PERSECOND FULL
But given that an object travels 2π rad in one full rotation, the angular velocity can be calculated using the following formulas: It is a measurement of how fast an object’s angular position changes with time, and by extension, how fast an object rotates around a point.Īngular velocity is mathematically defined as the angle that an object has travelled in radians, divided by the amount of time that has passed in seconds ( ω = θ/s). The angular velocity of an object, denoted by the symbol ω, is the rate of change of angle. When an object moves in a circular path, we measure its velocity in terms of angles, rather than the linear displacement. It is related to the period by the formula f = 1 / T. Frequency ( f): The frequency is the number of full rotations that an object completes in 1 second.Period of rotation (T): The period is the time taken for an object to complete a full rotation around a point of reference.When converting degrees to radians, remember that π rad = 180°. Radians (rad): This is typically the unit of choice for measuring angular displacement, with respect to motion in a circle.Using concepts of angular velocity, and centripetal acceleration & force, we can then calculate how fast the wheel is going in a circle, and how fast the car is moving.īefore going into the concepts proper, there are a few terms that you need to understand. Studying the way that objects undergo motion in a circle thus has several real-world applications.įor example, have you ever wondered how a car speedometer calculates the speed of the car? What it is measuring is not the amount of distance travelled, but rather the number of rotations that a wheel is making in a fixed amount of time. You can find formulas derived for each parameter below the calculator.Circular motion is everywhere around us, from the spinning of the wheels on your bicycle to the fans in your living room. That's why the calculator allows different units for each parameter and takes care of converting them to SI units and back. While the formula is not very hard, you can easily make errors by using wrong units, for example, revolutions per second instead of radians per second, centimeters instead of meters, and so on. If the cord’s length is 50 cm, what is the centripetal force? Example of such problem: A 500-gram ball, attached to the end of a cord, is revolved in a horizontal circle with an angular speed of 5 rad/s. Thus three parameters should be given in the problem statement, sometimes indirectly, and the fourth parameter should be calculated. If you have a problem with numeric data, you are supposed to use centripetal force formula: The calculator below can be used to verify solutions of centripetal force problems.


 0 kommentar(er)
0 kommentar(er)
